Basic Theory

The basic rule of an aircraft engine is that each cubic inch of displacement produces roughly 0.5 horse power. But I have rarely seen how this number is calculated. So that is the purpose of this post. We will walk through the math so that we can see how the thermodynamic calculations lead to that answer, and what other factors affect the power output.

We will limit our discussion to reciprocating four-stroke engines. So this is not applicable to turbines and jets. For an engine spinning at an rpm of f , the weight (W_a) of the air intake per minute can be written as:

W_a = D \times \frac{f}{2} \times \rho\hspace{2cm}(1)

where D is the displacement volume of the engine and \rho is the density of air. The \frac{f}{2} accounts for the fact that only one half of the cycles breathe air in. In other words, at 2700 rpm, each cylinder will have 1350 suction (and compression) cycles per minute. The other 1350 are combustion (and exhaust) cycles.

Next, let’s add fuel to the air. This is typically done with a carburetor or a fuel injector. The stoichiometric ratio of air to Avgas fuel is 14.7:1 by weight. Other fuels will have different ratios. Let’s represent this ratio with the symbol S_r. Combustion at the stoichiometric ratio produces the most amount of energy. In practice, this is not the best operating point because it can make the engine run too hot and cause cylinder head damage. Engines are often operated with less fuel (lean of peak) or with more fuel (rich of peak) to reduce temperatures. However, lets just stick to the stoichiometric value for the purpose of this calculation.

The weight of fuel added per unit time to the air to maintain the correct stoichiometric ratio becomes

W_f = \frac{W_a}{S_r}.\hspace{2cm}(2)

Next, we need to consider the energy released during combusion. This depends on the type of fuel. For gasoline (including Avgas), stoichiometric combustion results in 43 MJ/kg ( 14.8\times 10^6 ft-lb/lb). Let’s represent this value as Q_m. Therefore, the energy released from combusion per unit time (which we will write as P_t) will become

P_t = \frac{W_a}{S_r} \times Q_m.\hspace{2cm}(3)

P_t is the total power released as heat. Only a small portion of that will perform mechanical work. This depends on the heat engine efficiency, which we will write as \eta. It’s value can vary quite a bit, but most reciprocating internal combusion engines have values on the order of 25-30%.

Therefore, the crankshaft output power, P_c, becomes

P_c = \eta\times \frac{W_a}{S_r} \times Q_m.\hspace{2cm}(4)

Combining everything together, we can write this as

P_c = \eta \times D \times \frac{f}{2} \times \rho \times Q_m \times \frac{1}{S_r}. \hspace{1cm}(5)

The density of air at sea level is 0.0765 lb/cubic ft, or 4.4\times 10^{-5} lb/cubic inch. Assuming 2700 rpm, S_r=14.7, Q_m=14.8\times 10^6 ft-lb/lb, \eta=28\%, we can calculate the power from one cubic inch of displacement as follows:

P_c = 0.28 \times 1 \times \frac{2700}{2} \times 4.4\times 10^{-5} \times \frac{14.8\times 10^6}{14.7}=16,745 ~~{\rm ft\cdot lb/min.}

One hp is equal to 33,000 ft-lb/min. Therefore, 16,745 ft-lb/min is equivalent to approximately 0.5 hp/cubic inch of mechanical power, as expected. It is important to note that this value is valid only at 2700 rpm. If the rpm is lower, the power will be lower. Similarly if the rpm is higher, the power will be higher. But direct drive aircraft engines are typically limited to 2700 rpm due to propeller limitations. Therefore, 0.5 hp/cubic inch is a widely accepted number in aviation.

Fuel consumption is also easy to calculate. Using W_f = \frac{W_a}{S_r}, we can get

W_f = D \times \frac{f}{2} \times \rho\times \frac{1}{S_r}. \hspace{2cm}(6)

Using the same values as before, this works out to 0.004 lb/min, or 0.24 lb/hr for each cubic inch. The density of Avgas is 5.97 lb/gal. Therefore, we get a fuel consumption of 0.04 gal/hr/cubic inch of displacement. Again, this is true only when the rpm is 2700.

Additionally, the power output per unit weight of fuel is also easy calculate. If we divide crankshaft power P_c by the weight of the fuel per minute W_f,

\frac{P_c}{W_f} = \eta \times Q_m. \hspace{2cm}(7)

Using \eta=28\%, Q_m=14.8\times 10^6 ft-lb/lb, this works out to 4.14\times 10^6 ft-lb/lb. Dividing by 33,000 ft-lb/min gives 125.5 hp/lb/min, or 12.5 hp/gal/hr. This value is independent of rpm.

O-320 & O-540 engines

Automotive Engines

Automotive engines have smaller displacements, but run at higher rpm. A 2.5 liter (152 cubic inch) toyota engine is rated for a maximum of 6600 rpm. The heat engine efficiency is slightly higher due to electronic ignition controls and greater optimization. The combusion energy of autogas is nearly the same as Avgas. Assuming \eta=30\%, if we repeat the above calculation, we can get 200 hp. This value is equivalent to 1.3 hp/cubic inch, which is 2.5 times greater than the power density of a direct-drive aircraft engine. Again, this emphasizes the fact that 0.5 hp/cubic inch is specific to 2700 rpm.

Rotax 912 Engine

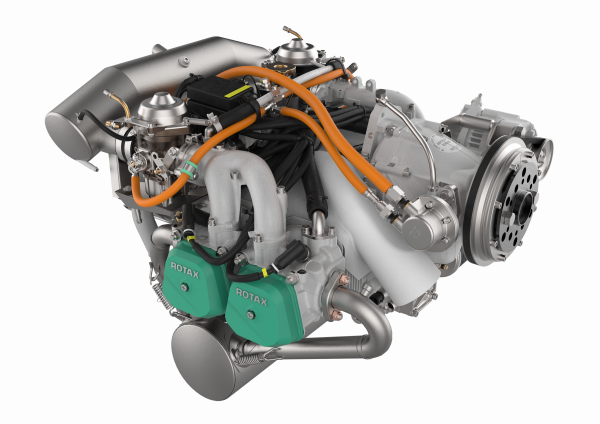
The Rotax 912 is an aviation engine, but it is designed to run at 5800 rpm similar to an automotive engine. In order to drive a propeller at 2700 rpm, it uses a reduction gear. For the purposes of the engine power, 5800 rpm is what matters. Just like with the automotive engine, the higher rpm allows for a larger power output per cubic inch. The displacement of the Rotax 912 engine is 83 cubic inches (about 3.8 times smaller than the O-320). The heat engine efficiency of the Rotax is a bit higher, at around 31%. The power output works out to 1.2 hp/cubic inch. This value is more than double the power density of the direct drive engines such as the O-320. The fuel flow rate is 0.087 gal/hr/cubic inch. The total performance of the Rotax 912, therefore, is 83 \times 1.2 = 100 hp, and the fuel flow is 83 \times 0.087 = 7.2 gal/hr. These values are consistent with the specifications of the engine.

Throttle Valve

In the above calculations we have assumed that the density of the intake air is the same as the outside air. This is true only when the throttle valve is fully open. So, what happens when we slowly close the throttle valve? Closing the throttle valve will cause the pressure of the intake air to decline. This is the pressure indicated by the manifold pressure gauge. Hence, the density \rho will also decline. Clearly, the outside air density remains unchanged. The blocking effect of the throttle plate creates a partial vacuum, which results in a lower pressure and lower density entering the cylinders. As \rho declines, the power output will decline. Assuming a fixed load, this will cause the rpm to decline. The decline in rpm will cause a further decline in power. This is a runway condition that can lead to the engine coming to a halt. The same thing can happen when the throttle is opened. The rpm will increase, which will increase the power and lead to dangerously high rpm values. In practice, however, loads do not remain constant with rpm. They typically increase with rpm. This will prevent the runaway conditions and stabilize the operating point. We will discuss this later in the discussion on propellers.

Power vs rpm

As indicated by equation (5), the power will scale linearly with rpm at a constant manifold pressure. But it does not continue indefinitely. The power will reach a maximum, and then start declining at higher rpm values. This is shown in the adjacent figure. This is due to increasing friction losses and lower combustion efficiencies at higher rpm. This is usually the maximum permissible rpm of the engine. Similarly, power vs manifold pressure at a fixed rpm will also show a linear relationship. Manifold pressure is normally limited to atmospheric pressure, but in turbo boosted engines, it can go higher.

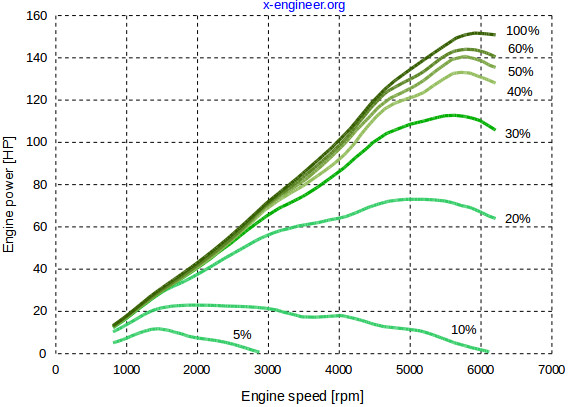
It’s also worth noting that it is possible to run the engine at higher rpms and higher powers than it is rated for, but it will most likely result in permanent damage. For example, if the load is too light and the throttle is fully open, the rpm can exceed the maximum limit.

Torque vs rpm

Torque is the rotational force imparted by the crankshaft. It is related to power and rpm as follows:

P_c = T \times f. \hspace{2cm}(8)

Comparing this to equation (5), we can see that torque can also be expressed as

T = \eta \times D \times \frac{1}{2} \times \rho \times Q_m \times \frac{1}{S_r}. \hspace{1cm}(9)

All of these parameters are nearly constant at a specific throttle setting, which means torque should be constant regardless of rpm or power.

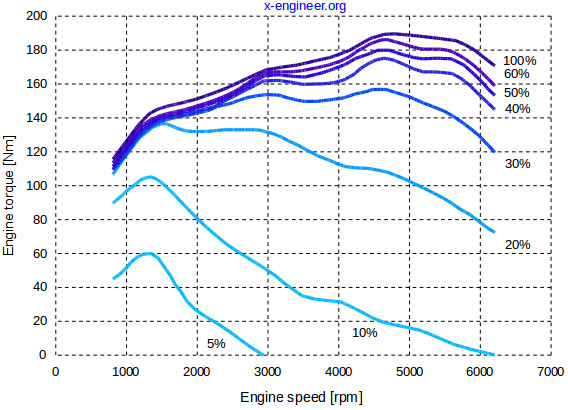
In practice, that is not exactly true. Torque increases slowly with rpm, though not as linearly as power increases with rpm. It reaches a maximum and then declines again.

Aircraft vs Automotive Engines

As we previously calculated, the power density of direct drive aircraft engines is about 0.5 hp/cubic inch. Automotive engines have much higher power densities. However, there are other reasons why direct drive engines are used in aircraft. High rpm engines require a reduction gear (transmission). This adds weight and complexity. Direct drive engines do not need a transmission. As a result, they are actually lighter despite their lower power density. They are also more reliable because there are fewer moving parts. Additionally, the lower power density of direct drive engines allows is to be air cooled. Higher power densities require liquid cooling, which requires additional components and additional weight.

Instead of volumetric power density, we can also calculate the power to weight ratio. The dry weight of an O-320 engine is 270 lb including all accessories. That gives a power to weight ratio of 0.6 hp/lb. The O-540 weighs 390 lb, and the power to weight ratio is 0.7 hp/lb. The Rotax 912 weighs 125 lb including the reduction gear, which translates to 0.8 hp/lb. Automotive engines rarely specify weight, but they are typically significantly heavier.

The Rotax engine includes a reduction gear and liquid cooling without a significant compromise in weight or reliablity. As an added benefit, the higher rpm allows unleaded lower octane automotive fuels to be used in Rotax engines. Direct drive engines running at lower rpm need additives to increase the octane rating to prevent engine knocking.

Summary

So, here are the takeaways from this analysis:

• Shaft power output is a product of intake air density (which is controlled by manifold pressure) and rpm. It does not matter if it is a constant speed prop or fixed pitch prop – power is the product of these two quantities.
• Direct drive engine are often limited to 2700 rpm. At this rpm, the power density works out to 0.5 hp/cubic inch of displacement.
• Automotive engines and Rotax engines use a gear reduction, allowing the crankshaft to spin faster. This allows the power density to be higher. In the Rotax 912, at 5800 rpm, the power density is 1.2 hp/cubic inch.
• The efficiency of most engines is about 30%. The energy content of most gasolines is about 20MJ/lb. The density of most gasolines is about 6 lb/gal. Combining all of these, we can say that 1 gal/hr of fuel flow should produce about 12.5 hp of shaft power.

It’s important to point out that crankshaft power is not the same as propulsion power. The propeller efficiency factors into how the crankshaft power is converted into propulsion power. We will look at this in the next article.